Quantifying Genetic Variation in Populations

 Consider a single locus with two alleles A & a:
    Let frequency of A allele f (A) = p
    Let frequency of a allele f (a) = q
    p & q are allele frequencies
    What are the genotype frequencies of AA, Aa, & aa?

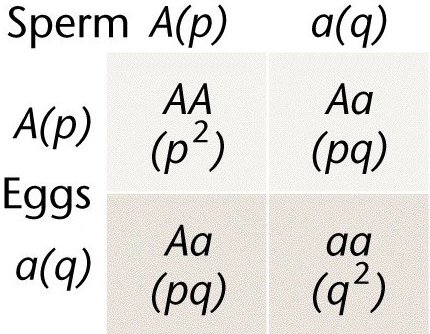
So:  f (AA) =    f(A) x f(A)  =  p2
        f (Aa)  =  [f(A) x f(a)] + [f(a) x f(A)]  =  pq + qp  =  2pq
        f (aa)  =    f(a) x f(a)  =  q2

Expected genotype frequencies can be calculated from allele frequency data:
 
 
Frequency of A & a alleles
Allele
A
a
Frequency
0.7
0.3
Formula
p
q

 
Frequency of pheno- & genotypes
Phenotype
AA
Aa
aa
Genotypes
AA
Aa
aa
Formula
p2
2pq
q2
Math
(0.7)2
(2)(0.7)(0.3)
(0.3)2
Frequency
0.49
0.42
0.09


The math can be extended to multiple alleles per locus

        For the human ABO blood group locus with three alleles, we know:
 
Frequency of I - locus alleles
Allele
IA
IB
 IO
Frequency
0.4
0.1
0.5
Formula
p
q
r

 
Frequency of ABO blood types
Phenotype
A
B
AB
O
Genotypes
IAIA & IAIO
IBIB & IBIO
IAIB
IOIO
Formula
p2 + 2pr
q2 + 2qr
2pq
r2
Frequency
0.56
0.11
0.08
0.25


The proportion of heterozygotes (H) at a locus is a measure of genetic variation

     For a locus with two alleles
         Ho = f(Aa) = observed heterozygosity
         He = 2pq  = 2q(1-q)  = 1 - q2  expected heterozygosity

         He is the probability that two alleles chosen at random from the population will be different

    For a locus with three  alleles
          Since     p + q + r = 1
          The trinomial expansion gives     (p + q + r)2 = p2 + 2pq + q2 + 2qr + r2 + 2pr = 1

          He = 2pq + 2pr + 2qr = 1 - (p2 + q2 + r2)

    In general, for a locus with multiple alleles
                      n
       He = 1 - (qi)2      for n alleles
                     i=1

        where qi = freq. of i th allele of n alleles at a locus
                In the ABO case:   He = 1 - (0.42 + 0.12 + 0.52) = 0.42

All text material ©2002  by Steven M. Carr