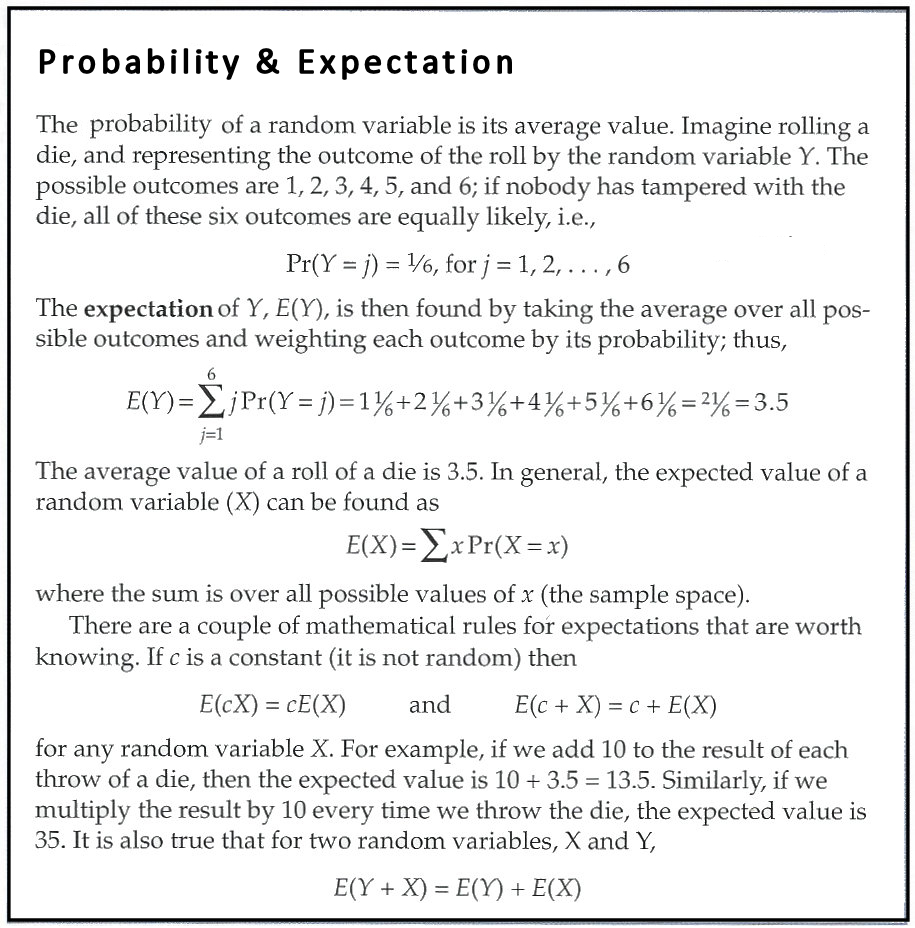
Expectation vs Probability

    When a single die is rolled, the outcomes 1, 2, 3, 4, 5, & 6 all occur with equal probability. For example, the probability of a 2 is Pr(Y = 2) = 1/6. Thus, over a long series of rolls, each value will occur an equal number of times. The calculation shows that the expectation of this series is E(Y) = 3.5: this is not a value that can be obtained on any single role of the die. Likewise, when a single coin is flipped, the outcomes H and T are equally probable, so Pr(Y = X) = 1/2. Once again, "half a head" is not an observable outcome, but over the long run E(Y) = 0.5, and we expect "50% Heads".

    Probability and average are the same, if and only if 'average' is limited to 'arithmetic mean' as in the example. The expectation of a variable might be the mode of its distribution, and the mode will not equal the mean if the distribution is skewed. The expectation of a complex function such as the Poisson Distribution is conditional on the occurence estimated.

    HOMEWORK: repeat the calculations of the probability distribution and expectation for two dice.